When we are asked to add a set of given numbers, we simply apply the formula of addition to it and derive the results. It is an easy task to calculate the small addition problems. What if the addition problem is big? What if we reach our limits of calculation?
There is a separate method in mathematics where we can solve big addition problems, even to the limits of infinity. Integration is a method that is used to find a summation under an expansive scale. The method is associated with a branch of mathematics called calculus. Integration is one of the two major kinds of calculus. The other kind is called differentiation. Even though conceptually, the level of the topic is very high, we will try to learn it schematically.
Before we begin solving big summation problems, we must understand numbers. It is the basis of all mathematical problems.
Let us understand integration from the ground up before we begin to learn different formulas and methods of integrating fractions or algebraic expressions.
What is Integration?
Calculating to find the area, volume, or displacement with definite values is an easy task. When the given values vary at different points and we discover a collection of small data that cannot be measured singularly, we bring in integration. In other words, integration could be defined as a process of adding the slices to make it whole. It is achieved through integrals. It assigns numbers to the function that derives the area, displace, volume, and other mathematical concepts that arise by combining infinitesimal data. Integration could also be described as the calculation of integrals. It is one of the two major kinds of calculus — differentiation and integration.
It is also called the reverse process of differentiation, where the function is disintegrated into parts to find the derivatives. Whereas integration, on the other hand, is used to find the antiderivatives of a function. Both the processes which constitute calculus are inverse of each other. However, the method of differentiating and integrating a function is linked through a theorem called the Fundamental Theorem of Calculus.
To represent the integration of the integral, with respect to x of a function, with real value f of a real variable x, the integral sign ∫ is denoted. To indicate the variable of integration, the symbol dx is used, which is also called the differential of the variable x.
The real-life application of integration includes calculation of the centre of gravity and the centre of mass, and it also helps to predict the position of the planets.
Based on the process that we are using to find the integrals of some functions, there are various types of integration to make the process of evaluation easier.
Types of Integration

Before we start exploring the different types of integration, we have to learn a bit more about integrals.
An integral is the fundamental object of calculus that corresponds to the addition of infinitesimal objects to find the function. It can also be interpreted as an area or the generalization of an area.
We can find two forms of integrals:
- Definite integrals: It comes with the limits of integration. It has the lower and upper limits as the values for the interval of integration. It also does not have any constant of integration.
It is represented as ∫ab f(x) dx
- Indefinite integrals: It has no limit for integration, and it contains an arbitrary constant.
It is represented as: ∫f(x) dx = F(x) + C
where C is a constant and f(x) is called an integrand.
You can learn more about integrals here.
Now, let’s continue understanding the different types of integration to make our large-scale summation problem easy.
Integration through substitution
Even though integration allows us to calculate a large sum, sometimes, we really find it hard to derive the integration of a function. However, there is a solution to that. We can introduce a new and freestanding variable to find the integration.
In the provided integral function ∫f(x), we can transform it by substituting the independent value of x into t.
For example,
After substituting the value of independent variable x as g(t) in the given integral function that is, ∫f(x),
We get, dx/dt = g’(t)
We can also write, dx = g’(t).dt
Therefore, after substituting the above independent variable, we get,
I = ∫f(x).dx = f(g(t)).g'(t).dt
Integration through parts

It is a special kind of integration method where the function that is to be integrated — the integrand f(x) — is the product of two or more than two functions.
Consider an integrand function as f(x).g(x)
It will be mathematically depicted as;
∫f(x).g(x).dx = f(x).∫g(x).dx – ∫(f′(x).∫g(x).dx).dx
It can be denoted as,
Integral of the product of any two functions = (First function × Integral of the second function) – Integral of [(differentiation of the first function) × Integral of the second function]
One can follow the ILATE rule for deciding the first and the second functions.
ILATE rule – This rule helps to decide the term that should be differentiated first and which term to be integrated first. The term closer to the first letter is differentiated first, and the term closer to the last letter is integrated first.
I – Inverse
L – Logarithm
A – Algebraic
T – Trigonometric
E – Exponential
Integration through trigonometric identities
The trigonometric identities are used with the integrand involving certain trigonometric identities during the process of integration. This is done to streamline the function so that it is integrated easily.
Out of many trigonometric identities, a few are mentioned below:
- Sin2x = (1 – cos 2x) / 2
- Sin3x = (3 sin x – sin 3x) / 4
- Cos3x = (3 cos x + cos 3x) / 4
- Cos2x = (1 + cos 2x) / 2
Integrating particular functions
There are some other forms of integration that require certain integration formulas to turn them into standardized forms of the integrand. By using the direct form of integration method, these standardized integrands can easily be derived.
Below are six important formulas:
- ∫ dx / (x2 – a2) = ½ a log | (x – a) | + C
- ∫ dx / (a2 – x2) = ½ a log | (a + x) / (a – x) | + C
- ∫ dx / (x2 + a2) = 1/a tan-1 (x/a) + C
- ∫ dx / √(x2 – a2) = log | x + √(x2 – a2) | + C
- ∫ dx / √(a2 – x2) = sin-1 (xa) + C
- ∫ dx / √(x2 + a2) = log | x + √(x2 + a2) | + C
Integration through a partial fraction
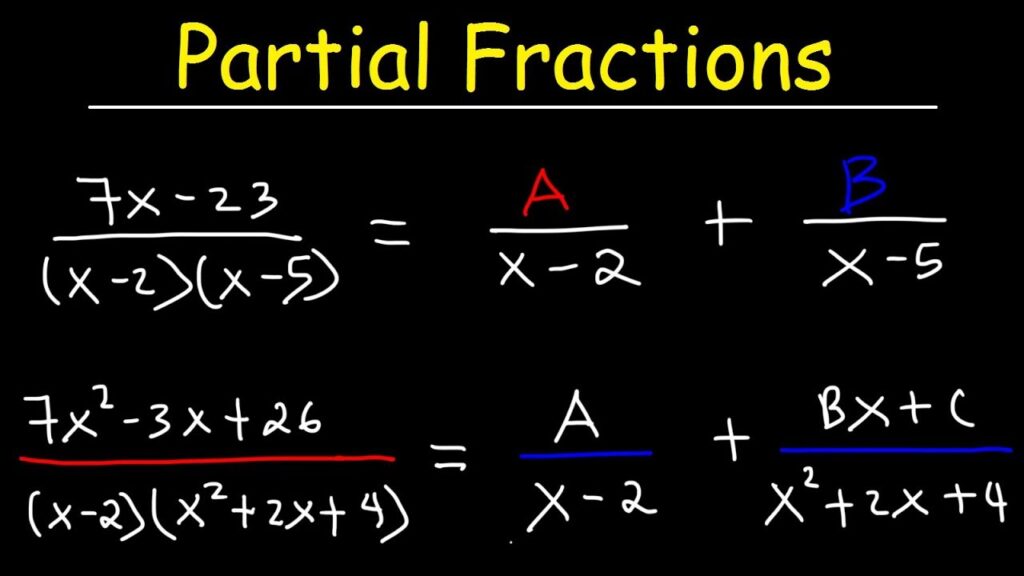
As we know that we can represent a rational number in the form of p/q, where p and q are integers, and the value of the denominator q is not equal to zero.
Likewise, a rational fraction can also be represented as the proportion of two polynomials, and it can be denoted as a partial fraction, p(x)/q(x), where the numerator p(x) should not be equal to zero.
Generally, we can find two kinds of partial fraction; Proper partial fraction and improper partial fraction.
- Proper partial fraction: It is called a proper partial fraction when the numerator’s degree r is less than the denominator.
- Improper partial fraction: If the degree of the numerator is greater than the degree of the denominator, then the partial fraction will be called an improper partial fraction. Therefore, the fraction can be further simplified into parts to be integrated easily.
An improper fraction can be reduced to a proper fraction by the long division process. The following is an example of integration by a partial fraction:
Suppose, we want to evaluate ∫ [P(x)/Q(x)] dx and P(x)/Q(x) is a proper rational fraction. By using partial fraction decomposition, we can write the integrand as the sum of simpler rational fractions. After this, we can carry out the integration method easily.
Partial fraction decomposition – It is a process consisting of expressing the fraction as a sum of a polynomial and one or several fractions with a simpler denominator.
Important Formulas for Integration
The following are the commonly used formulas used to solve problems related to integration.
- ∫ a dx = ax + C
- ∫ 1 dx + x + C
- ∫ sin x dx = -cos x + C
- ∫ xn dx = xn+1 / n+1 + C; n ≠ -1
- ∫ sec2 x dx = tan x + C
- ∫ cos x dx = sin x + C
- ∫ sec x .tan x dx = sec x + C
- ∫ cos x cot x dx = sec x + C
- ∫ ex dx = ex + C
- ∫ ax dx = ax/ln a + C; a > 0, a ≠ 1
- ∫ 1/√1-x2 dx = sin-1 x+C
- ∫ 1/1+x2 dx = tan-1 x+C
- ∫ (1/|x|)√x2 – 1 dx = sec-1 x + C
- ∫ sinn (x) dx = -1/n (sin)n-1 (x) cos(x) + n-1/n ∫sinn-2 (x)dx
- ∫ cosn (x) dx = i/n (cos)n-1 (x) sin(x) + n-1/n ∫cosn-2 (x)dx
Now that you know the basics of integration and its various types, head over to Cuemath to learn more and stay ahead!